EQUATION OF CONTINUITY
So today we are going to talk about a very important and interesting concept of hydrodynamics i.e equation of continuity.
Ask yourself this question:
What is the mean of continuity? And why are we using it here in hydrodynamics?
Continuity means anything which covers equal displacement in equal time interval.
In fluid mechanics, if V volume of liquid is entering in ∆t then same volume of liquid is exitting in ∆t also.
Fluid has so many properties, but the property by which it became unique, is the property of incompressible, it means fluid can’t be compressed by any means,
Here fluid means liquid,
In hydrodynamics we consider a ideal fluid, because mathematical modelling of real fluid is very complicated.
A ideal fluid is that fluid which is non viscous, incompressible and irrotational.
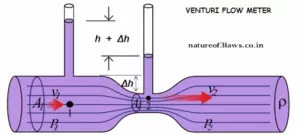
So it is observed that when a ideal fluid flows through the pipe of different cross sectional area, the volume of fluid which is entering is equal to the volume of fluid which is exit. If fluid is ideal then it’s density is constant at every point.
If volume is equal and it’s density is constant then mass of fluid which is entering is also equal to the mass of fluid which is coming out.
V = m1/ρ = m2/ρ then m1 = m2
Conservation of mass is applying here.
DERIVATION FOR EQUATION OF CONTINUITY
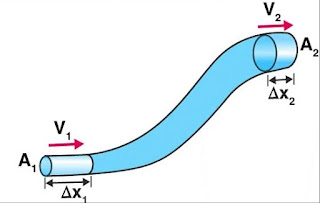
Let’s take a pipe of different cross sectional area , with one end have A2 , v2 ,∆t ,P2 , ρ2 and at second end A1 , v1 , ∆t , and P1 , ρ1.
Here ∆t is small time interval in which fluid get displaced. And ρ1 =ρ2 due to ideal nature of fluid.
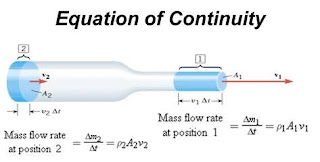
We know that mass of fluid which is entering is equal to the mass of fluid which is coming out.
So. m2 = m1
And m2 = Vρ2 , and m1 = Vρ1. Then we can say that
Vρ2 = Vρ1
Then , Volume is given as
V =cross sectional area × displacement
So ,. V = A2v2∆t and V = A1v1∆t
Putting value we get
A2v2∆tρ2 = A1v1∆tρ1. ( But ρ1 =ρ2)
So, we get after cancelling ∆t and ρ both side
V = A1v1 = A2v2 = constant
This equation is called the equation of continuity. Watch this video for more reference.