SPREAD TO HELP OTHERS
Today we are going to talk about the various types of pendulums and their time periods. Pendulums plays a very important role in simple harmonic motion physics that is also called oscillation. So for everyone who read and understand physics, it is necessary to understand the underlying concept of pendulums. So here we are going to cover a detailed talk on various types of pendulums. So stay tuned with us till end.

So let’s starts…
First of all we have to understand that, what is pendulum? Pendulum is derived from a Latin word ‘pendulus’ which means hanging.
So, A pendulum is nothing but it is only a arrangements in which, when a weight is suspended from a pivot with a inextensible cord such that it can swing freely back and forth after applying a small force on the weight.
When no any external force is applied in the bob, then pendulum remains in rest position. And the position where it remains in rest is called mean position. When a pendulum is swinging back and forth from his mean position then it get displaced, and we see that after some time it comes in rest(mean position) Then from this we can conclude that mean position is applying some inward force ,it is when, when bob swings away from the mean position. And this inward force is called restoring force. It means we can say that always a restoring force is applied by the mean position on the bob , by which it comes in rest. And this restoring force is always directly proportional to the displacement of the bob from the mean position. And this type of back and forth motion of pendulums in which restoring force which is applied by the mean position, is always directly proportional to displacement from the mean position is called oscillation or simple harmonic motion.
All the pendulums which we are going to read, perform SHM, means oscillates about their mean position.
We are going to talk about four types of pendulums, which are listed below:
1). Simple pendulum
2). Compound or physical pendulum
3). Conical pendulum
4). Torsional pendulum
SIMPLE PENDULUM

A simple pendulum is also called simple Gravitational pendulum, it is idealised simple pendulum consist of a weight(bob) which is suspended by a inextensible and massless cord from a pivot having zero air drag and friction. If small force is applied on the simple Gravitational pendulum , it will start oscillating with constant amplitude. But when Normal pendulums or real pendulums start oscillating back and forth, it motion is hindered by the air drag and friction , by which it’s amplitude gradually declines.
Practically it is not possible to make a ideal simple pendulum. So for practically uses , a simple pendulum is obtained by suspending a weight(bob) with a fine cotton thread from a pivot.
DERIVATION FOR TIME PERIOD OF A SIMPLE PENDULUM

We have to understand the meaning of time period , time period is nothing but it is only the time taken by the bob to complete one full oscillation.
Now, consider a ideal simple pendulum, having bob of mass m , and hangs with a massless and inextensible cord of length L , and other end of the cord is fixed to a pivot , as shown above.
Now, think that this pendulum is swinging back and forth and you stop it at any instant of oscillation as shown above.
At any instant of stoppage of the pendulum, we find that θ is the angular displacement which is made by the bob from the mean position.
W = mg is the weight which is acting towards the ground and balance by the normal reaction R,
R = W = mg, and θ is the angle between the cord and the normal reaction.
After resolving the mg in X and Y components, we get -mgsinθ along X axis and -mgcosθ along Y axis.
-mgsinθ act as restoring force, because during oscillations this force pull the bob towards the mean position. Minus sign is because this force acts in opposite direction of the motion of the bob. And -mgcosθ is acted along the cord but in the opposite direction of the tension in the cord. T = -mgcosθ ,. and restoring force = -mgsinθ
Restoring force (F) is perpendicular to the length of the cord(L),
And we know that torque τ is r × F = rFsinθ , here r is length L and force is mgsinθ.
Then τ is given as mgsinθL ,
τ = -mgLsinθ = Iα , where I is moment of inertia and α is angular acceleration.
If θ is very small then sinθ = θ
τ = -mgLθ =Iα , then ,
Iα = -mgLθ
α = (-mgL/I)θ , equating with a = -ω^2x
-ω^2 = -mgL/I. (minus minus get cancelled)
ω = √(mgL/I)
We know that ω = 2π/T then we get,
2π/T = √(mgL/I)
T = 2π√(I/mgL)
Moment of inertia of bob is mL^2 , putting it into a above expression , we get,
T = 2π√(mL^2/mgL)
T = 2π√(L/g)
This is the required time period of the simple pendulum.
But this formula is not general, the general formula for time period of simple pendulum is
T = 2π√RL/g(R+L) , where R is the radius of the earth and L is the length of the pendulum.
RESULTS TO BE NOTED
*. If angular amplitude of the simple pendulum is more , then the time period is =
T = 2π√(L/g)×(1+θ^2/16) , where θ is in radians.
*. On increasing length of simple pendulum, time period increases, but time period of simple pendulum of infinite length is 84.6 minutes, which is maximum and is equal to the
T = 2π√R/g
*. If time period of clock based on simple pendulum increases , then click will be slow , but if time period decreases then clock will be fast.
*. If g remains constant and ∆l changes in length, then , (∆T/T)×100 = (∆l×100/2l)
*. If l remains constant and ∆g changes in acceleration, then , (∆T/T)×100 = -(∆g×100/2g)
*. If both length and acceleration is changes by ∆l and ∆g then,
(∆T/T)×100 = 1/2[(∆l/l)-(∆g/g)]×100
Now we are going to talk about physical pendulum or compound pendulum.
COMPOUND PENDULUM AND ITS TIME PERIOD
When a rigid body is suspended from one axis and made to oscillates about it , then this types of pendulums is called compound or physical pendulum. See below;


In this figure O is pivot point , Lc is the distance of the centre of mass C from the pivot. And θ is the small angular displacement , which is made by the line joining the centre of mass to pivot and axis of mean position.
During the oscillation , for small angular displacement θ , restoring torque τ is given by;
τ = -mgLθ. [ Here Lc is taken as L]
Then , torque can be written as,
τ =Iα = -mgLθ
Then , α = -mgLθ/I , equating with a = -ω^2x
We get , ω ^2 = mgL/I [(minus minus get cancelled)]
ω = √mgL/I
We know that ω = 2π/T then we get,
2π/T = √(mgL/I)
T = 2π√(I/mgL), where I is moment of inertia.
Moment of inertia is given as I = Icm + mL^2
And Icm is given by mK^2 , where K is radius of gyration about axis passing from the centre of mass.
I = mK^2 + mL^2
Now putting all the values above , we get;
T = 2π√(mK^2 + mL^2)/mgL
T = 2π√(K^2 + L^2)/gL = 2π√Leq/g
Where Leq is K^2/L + L = equivalent length of the compound pendulum.
If L = K then Time Period is minimum, which is given as,
T = 2π√2K/g
This is required time period of compound pendulum.
Now we are going to talk about conical pendulum.
CONICAL PENDULUM
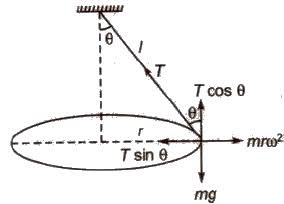
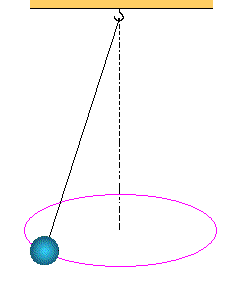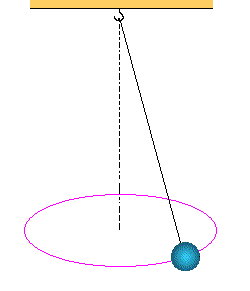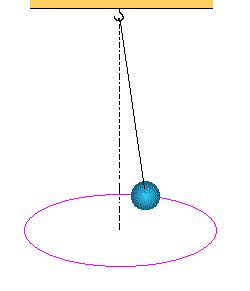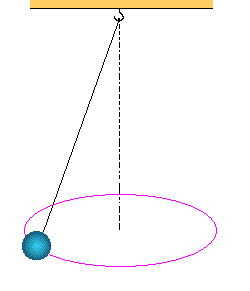
So what is a conical pendulum , A conical pendulum consist of a string whose one end is tied to a fixed point and other free end is tied with a Bob. When the bob is drawn aside and give a horizontal push , then it start describing a horizontal circular path with uniform angular velocity ω in such a way that it’s string made an angle θ with vertical. As the string traces the surface of a cone of semi vertical angle θ , then whole figure looks like a cone, due to this it is called conical pendulum.
For reference see below;

DERIVATION OF TIME PERIOD OF CONICAL PENDULUM

Let’s consider the T is the tension in the string and L is the length of the string, and r is the radius is the cone , which is made by the horizontal circular motion of the string. And h is the height of the cone. m is the mass of the bob.
After resolving the different forces on the bob in their components then, we find that;
Vertical component Tcosθ is balanced by the weight mg.
Tcosθ = mg ….(1)
And horizontal component Tsinθ is balanced by the centrifugal force mrω^2
Tsinθ = mrω^2…….(2)
And cosθ is equal to h/L and sinθ is equal to r/L
cosθ =h/L, and h = Lcosθ and r = Lsinθ
Dividing equation (2) by equation (1) we get,
tanθ = rω^2/g
…… ω^2 = gtanθ/r
…….. ω = √gtanθ/r
Putting r = Lsinθ in place of r , then we get,
ω = √gtanθ/Lsinθ
…… ω = √g/Lcosθ … and Lcosθ = h
Then. ω = √g/h
ω = 2π/T = √g/h
Then……. T = 2π√h/g
This is the required time period of conical pendulum.
Now we are going to talk about torsional pendulum.
TORSIONAL PENDULUM AND ITS TIME PERIOD

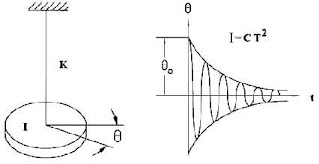
First of all , we have understand the meaning of torsion. Have you ever twist any flexible grass or any rubber objects. What do you see, you must have seen that after twisting it many times it again comes to its original condition, or it tends to come in its original position. Means when we applied a force to twist and after releasing the twisting force, a opposite force is applied to regain its original condition. This is called torsion , and the pendulum which works on this concept called torsional pendulum.
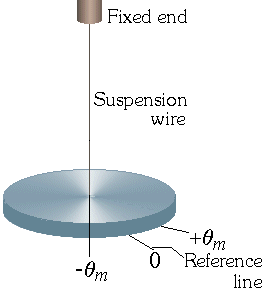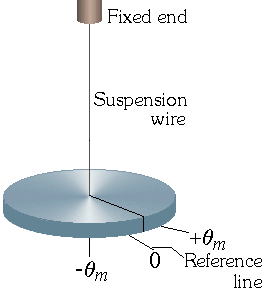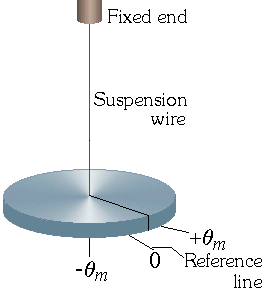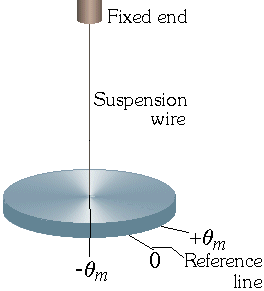

Restoring torque is directly proportional to the θ.
Where θ is angular displacement.
For more angular displacement we have to apply more force to twisting it.
τ = -Cθ. [C is the torsional constant]
Then ,. Iα = -Cθ
….. α = -Cθ/I. (where I is moment of inertia about vertical axis)
equating with a = -ω^2x ,.
We get -ω^2 = -C/I [(minus minus get cancelled)]
Then. ω = √C/I
….. ω = 2π/T = √C/I
Then ,….. T = 2π√I/C
This is required time period of torsional pendulum.
SPREAD TO HELP OTHERS