ALTERNATING CURRENT

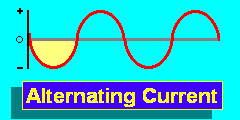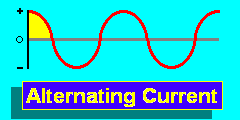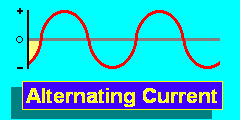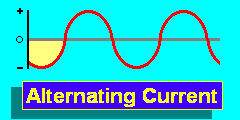
*. It is the current which varies in magnitude continuously and changes its direction alternatively and periodically.

It is represented as , I = I0sinωt , where I0 is the maximum value of AC current called as peak current. I is called the instantaneous value of alternating current. ω is called angular frequency of alternating current. Which is given as-

ω = 2π/T = 2πυ, T is time period of A.C and υ is frequency of A.C.

*. It is the type of sinusoidal waves , which are represented by sine and cosine angle.

*. Alternating voltage or EMF can be represented as. E = E0sinωt , where E is instantaneous value of alternating voltage , E0 is the peak voltage.

MEAN VALUE OF A.C

*. It is the value of alternating current , which send same amount of electric charge through the circuit in half cycle as it sent by steady current in the same time. It is denoted by Im. Derivations for mean value of alternating current over half cycle is as below.

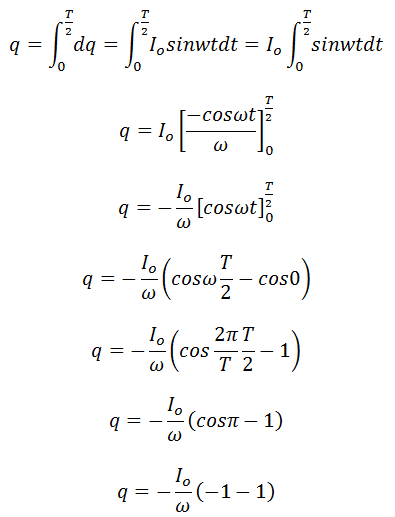
*. Mean value ofAlternating current over a complete cycle is zero.

*. Similarly Alternating EMF is given as-

Em = 2E0/π = 0.637E0

ROOT MEAN SQUARE VALUE OF AC.

*. It is the value of alternating current over a complete cycle which would generate same amount of heat energy in the circuit , as it is generated by steady current in the same circuit.

It is denoted as I.rms , I.eff or Iv.

Derivations for RMS current is as follow:

*. Similarly RMS voltage or EMF is given as-

E.rms = E0/√2 = 0.707E0 = 70.7% of E0.

*. Form factor is given as = I.rms/I.m = 1.11

AC THROUGH RESISTOR

*. Instantaneous EMF is E = E0sinωt

Then I = E/R = E0sinωt/R = I0sinωt

*. When AC flow through the resistor then voltage and current are in same phase.

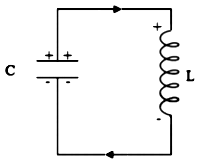
AC THROUGH INDUCTOR

*. The instantaneous voltage across the inductor is E = E0sinωt and I = I0sin(ωt- π/2)

Where I0 is given as = E0/ωL

*. In the inductor current lags behind the voltage by π/2 , and voltage lead the current by π/2.

*. INDUCTIVE REACTANCE – In electrical circuits inductor plays the role of resistor , when Alternating current passes through the inductor it opposes the flow of Alternating current , this resistance by the inductor is called the inductive reactance. It is denoted as XL.

*. Inductive reactance XL = ωL = 2πυL

AC THROUGH CAPACITOR

*. Instantaneous voltage across the capacitor is

E = E0sinωt and I = I0sin(ωt+π/2)

Where I0 is equal to ωCE0.

*. Current leads the voltage by the angle π/2 and voltage lags behind the current by the angle π/2.

*. CAPACITIVE REACTANCE – In the electrical circuits capacitor also plays the role of resistor. When AC passes through it , it opposes the flow of Alternating current , so resistance through the capacitor is called capacitive reactance.

It is denoted as Xc.

*. Capacitive reactance is given as , Xc = 1/ωC

= 1/2πυC

AC THROUGH R-L CIRCUIT

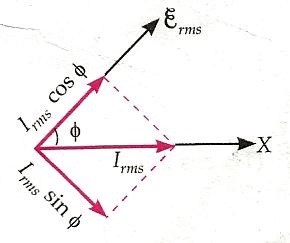
*. If AC source is E = E0sinωt and the instantaneous current in the circuit is

I = I0sin(ωt – φ) , where I0 is = E0/Z , where Z is the impedance of the L-R circuit.

Z = √(R^2+XL^2)

*. In this circuit voltage leads current from 0 to π/2.

*. Tanφ = XL/R and cosφ = R/Z

*. Resultant voltage is given as V= √(VL^2+VR^2)

[Here VL is voltage across inductor and VR is voltage across resistor]

A.C THROUGH R-C CIRCUIT

*. If AC source is E = E0sinωt and the instantaneous current in the circuit is

I = I0sin(ωt – φ) , where I0 is = E0/Z , where Z is the impedance of the L-R circuit.

Z = √(R^2+Xc^2)

*. In this circuit voltage lags current from -π/2 to 0.

*. Tanφ = Xc/R and cosφ = R/Z

*. Resultant voltage in the circuit is –

V= √(Vc^2+VR^2)

[Here Vc is voltage across capacitor and VR is voltage across resistor]

AC THROUGH L-C CIRCUIT

*. If AC source is E = E0sinωt and the instantaneous current in the circuit is

I = I0sin(ωt – φ) , where I0 is = E0/Z , where Z is the impedance of the L-C circuit.

Z = |XL – Xc|

*. If xL is greater than Xc then voltage leads current by +π/2. But if Xc is greater than xL then voltage lags by -π/2

*. Resultant voltage in the circuit is-

V = vL- Vc , if vL > Vc

V = Vc – vL , if Vc > vL

AC THROUGH L-C-R CIRCUIT

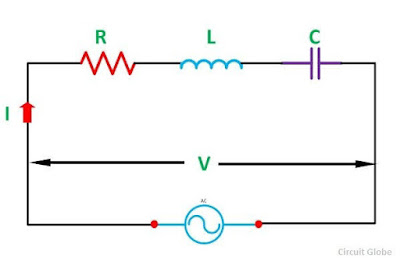
*. If AC source is E = E0sinωt and the instantaneous current in the circuit is

I = I0sin(ωt – φ) , where I0 is = E0/Z , where Z is the impedance of the L-C-R circuit.

Z = √[R^2+ (xL – Xc)^2] = √[R^2+ (ωL – 1/ωC)^2]

*. In this circuit Alternating current lags behind the voltage by phase φ.

Tanφ = (xL – Xc)/R and cosφ = R/Z

*. vL = I0.xL , Vc = I0.Xc , vR = I0.R

*. If xL = Xc , then tanφ = 0 and Z = R and voltage and current are in same phase. The AC circuit is resistive.

If xL > Xc, then tanφ is positive and voltage leads the current by phase angle Φ.

And circuit is called inductive dominant

If xL

And circuit is called capacitance dominant.

IMPEDANCE TRIANGLE

Impedance triangle is right angled triangle whose base is ohmic resistance (R) and it’s altitude is resultant reactance (xL – Xc) and it’s hypotenuse represent the impedance (Z).

Impedance Z = √[R^2+ (xL – Xc)^2]

= √[R^2+ (ωL – 1/ωC)^2]

ADMITTANCE – It is the reciprocal of the impedance of a AC circuit. It is denoted as Y.

Y = 1/Z = 1/√[R^2+ (xL – Xc)^2]

Unit of admittance is per ohm or Siemens.

SUSCEPTANCE – It is the reciprocal of the reactance of the AC circuit , and it is denoted by S.

✓ susceptance = 1/reactance

It unit is same as admittance as per ohm or Siemens.

*. Reciprocal of inductive reactance is called inductive susceptance.

sL = 1/ωL

Reciprocal of the capacitive reactance is called the capacitive susceptance.

*. Capacitive susceptance = 1/capacitive reactance

Sc = ωC

RESONANCE IN L-C-R CIRCUIT

*. An AC circuit is said to be resonant circuit, when the natural frequency of the circuit become equal to the frequency of the applied voltage.

*. At resonance

xL = Xc , vL = Vc

φ become zero [ V and I is in the same phase.]

Z(min) = R, because xL- Xc = 0.

I(max) = V/R

*. Resonant frequency

When xL = Xc then ωL = 1/ωC , ω = 1√LC

Or resonant frequency υR = 1/2π√LC

*. Bandwidth ∆υ = υ2 – υ1

*. QUALITY FACTOR (Q) – It gives the idea about the stored energy as well as lost energy. It measures the sharpness of the resonance of the L-C-R circuit.

Q = 2π×max energy stored per cycle/max energy lost per cycle.

*. Q = xL(r)/R = Xc(r)/R = 2π(υr)L/R = 1/2π(υr)CR

= √(L/C.R^2) = υr/bandwidth = υr/(υ2 – υ1).

*. Q- factor denotes the sharpness of the tuning.

– High Q factor means lower rate of energy loss.

– Higher value of Q- factor means sharper peak current.

ASSOCIATION OF POWER IN AC CIRCUIT

*. If AC source is E = E0sinωt and the instantaneous current in the circuit is

I = I0sin(ωt – φ) then instantaneous power in the circuit is –

P = E0sinωt.I0sin(ωt – φ)

= E0.I0sinωt.(sinωt.cosφ – cosωt.sinφ)

*. AVERAGE POWER (Pav)

ZI(max)^2 = V0.I0^2/I0 = V0.I0 , so it can be written as , V0.I0.cosφ/2

*.Root mean square power P(rms)= E(rms).I(rms)

*. Power factor cosφ = average power/rms power

Cosφ = R/Z

*. Maximum power dissipated in resistive circuit or L-C-R resonant circuit.

*. No power dissipated in purely inductive and Purley capacitive circuit. So current flowing through these circuit is called wattless current.

CHOKE COIL

*. It is the coil of high inductance and negligible resistance.

*. It is used to control the current in the AC circuit at minimum power loss. that is;

cosφ =r/Z = r/√(r^2 + xL^2) = (r/ωL)~0

*. Ideal choke coil has zero resistance.

*. Choke coil is the work of series L-R circuit. It choke coil have small resistance r then current is given by I = V/Z , Z = √[(R+r)^2 + (ωL)^2]

LC OSCILLATION

*. It is the oscillation of energy between capacitor and inductor. Capacitor produce electric field energy and inductor produce magnetic field energy.

*. The frequency of oscillation is υ = 1/2π√LC.

*. In the LC oscillation , according to Kirchhoff’s loop rule :

q/C – LdI/dt = 0 , but I = -dq/dt = – d^2q/dt^2

Then on solving we get-

d^2q/dt^2 + q/LC = 0 , comparing it with equation of simple harmonic motion

d^2x/dt^2 + ω^2x=0 , we get, ω^2=1/LC

Or ; ω = 1/√LC

*. Electrical energy stored in capacitor is

Uc = q^2/2C

*. Magnetic energy stored in inductor is

uL = LI0^2/2 , where I0 max. current

*. In the LC oscillation
U = q^2/2C =LI0^2/2

TRANSFORMER

*. It is a Device which is used to increase or decrease the Alternating current. Which is based on the phenomenon of mutual induction.

*. If transformer is ideal means there is no loss of energy in the transformer then input power is equal to output power.

P = V×I , Vp×Ip = Vs×Is

*. Constructing a ideal transformer is impossible, always some energy is escaped in the form of heat. 95% or more efficient transformer is regarded as best and we’ll designed transformer.

*. In any transformer