THE PROJECTILE MOTION

It is the type of motion in which if any object is fired with some initial velocity near the earth surface , then it’s motion follows a curved path called projectile motion. This curved path by the objects is called the trajectory of the projectile. Any missiles which follows these path called ballistic missiles.

SOME EXAMPLES

*. When we throw a ball.

* Firing of a missile.

*. Path of the water which is flowing from the hole of a tank.

*. Motion of a bullet. etc

SOME ASSUMPTIONS IN THE STUDY OF PROJECTILE MOTION

*. Zero air resistance.

*. Effect of earth rotation on the object is negligible.

*. Constant acceleration due to gravity.

EQUATION OF THE TRAJECTORY

MOTION IN HORIZONTAL DIRECTION

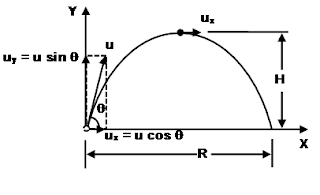
The velocity of the object in horizontal direction is constant, so it’s acceleration is zero.

The position of the object at any time t in the horizontal direction is-

x = x0 + (ux)t + 1/2(ax)t^2 ,

[ux and ax is horizontal velocity and acceleration]

But x0 = 0 , ux = ucosθ , ax = 0 , and t=t

Substituting these values in the above equation, we get.

x = ucosθ.t

t = x/ucosθ

MOTION IN VERTICAL DIRECTION

In the vertical direction object velocity starts decreasing due to acceleration due to gravity. So acceleration due to gravity is taken as (ay) = -g

The position of the object at any time t in the vertical direction is given as;

y = y0 + (uy)t + 1/2(ay)t^2

But y0 = 0 , uy = usinθ , ay = -g, and t = t

y = usinθ.t – 1/2gt^2

Substituting the value of t = x/ucosθ

y = usinθ( x/ucosθ) – 1/2g(x/ucosθ)^2

y = xtanθ – (gx^2/2.u^2cos^2.θ)

This is the trajectory of the object who is performing projectile motion.

*. Velocity of the object at any time t is given by-

v = √[u^2+(gt)^2 – 2ugtsinθ]

TIME OF FLIGHT

It is the total time for which a projectile remains in air. It is denoted by T.

Time of flight can be split in the two time format , one is time of ascent from O to H and second is time of descent from H to R.

Total time can be expressed as:

T = t+t = 2t , t = T/2

Takes a vertical upward motion of an object from O to H.

uy = usinθ , ay = -g , t = T/2 , and vy = 0, [vy become 0 at the maximum height of flight]

vy = uy + ay.t

0 = usinθ -g(T/2)

Time of flight T = 2usinθ/g

MAXIMUM HEIGHT

It is the maximum vertical height attained by the object during its flight.

Takes a vertical upward motion of an object from O to H.

We have,

uy = usinθ , ay = -g , y0 = 0 , y = H , t = T/2

Using second equation of motion.

y = y0 + (uy)t + 1/2(ay)t^2

Substituting above values; we get,

H = 0 + usinθ.(usinθ/g) + 1/2(-g).(usinθ/g)^2

H = (u^2.sin^2.θ/g) – 1/2(u^2.sin^2.θ/g)

Then H = u^2.sin^2.θ/2g

HORIZONTAL RANGE

It is the horizontal distance covered by the object during its flight. It is denoted by R.

If the uniform horizontal velocity of the object is ucosθ and it’s time of flight is T , then distance covered is-

R = ucosθ ×T = ucosθ×2usinθ/g = u^2.sin2θ/g

Range is maximum if sin2θ = 1 , sin(π/2) =1

It means , 2θ = π/2 , θ = π/4 = 45°

[NOTE: here θ is the angle between the velocity and the horizontal range, if the object is fired at the angle θ with the vertical, then use (β=90° – θ) angle with horizontal.]

MOTION OF THE PROJECTILE ON INCLINED PLANE

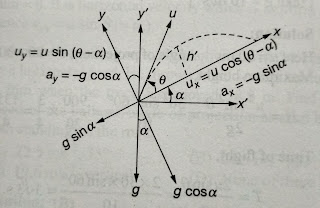
Take a plane of inclination α , and if projectile is fixed with angle θ with the horizontal range , then (θ – σ) is the angle of projectile with the inclined plane.

*. TIME OF FLIGHT

T’ = 2usin(θ – α)/gcosα

*. RANGE

R’ = 2u^2.sin(θ – α). cosθ/g.cos^2α

R’ = u^2[sin(2θ – α) – sinα]/gcos^2.α

Range is maximum if 2θ – α = π/2 or

θ – α = π/2 – θ

Then

R'(max) = u^2[1 – sinα]/gcos^2.α